Pascal's Triangle
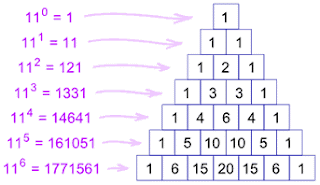
https://www.mathsisfun.com/pascals-triangle.html Fun fact: The sum of each row is an exponent of 11! Blaise Pascal was born on June 19, 1623, in Clermont-Ferrand, France. He was a mathematician, physicist, and religious philosopher. He is not just famous for creating Pascal's Triangle, he also created Pascal's principles of pressure, and he wrote a religious doctrine that instead of experiencing God through reason it should be through the heart. His principles influenced later philosophers including Jean-Jacques Rousseau and Henri Bergson. He most mainly impacted by his family. His father was a respected mathematician, and his sister was a literary prodigy. With his success in mathematics, he was able to help his father who was a judge in the tax court by creating the Pascaline, which was a machine that helped calculate taxes. This was the machine that really made him famous. For a while, he tested the theories of many later earlier physicist like Galileo, and Ev...