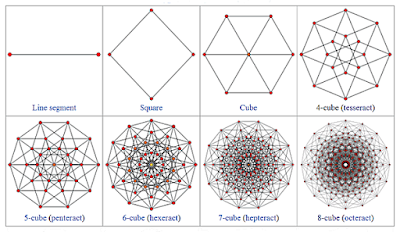
Hypercube, Tesseract

A tesseract is the 4-dimension of a cube and a hypercube is the n-dimension of a cube. A tesseract is to a cube as a cube is to a square. We will never know internally what a tesseract looks like because we are in the third dimension. To create a cube, you move a square one unit length perpendicular to the plane it lies on, and by doing this you create a three-dimensional cube. Then to create a tesseract, you move the cube one unit length into the fourth-dimension. Charles Howard Hinton was the first to coin the word tesseract, in 1888 in his book A New Era of Thought.

After watching many videos and reading a couple of articles, I have to say that tesseracts and hypercubes are such a hard concept to fully understand. I have a simple understanding of what a tesseract is, and how to create a tesseract. But at the same time, it is hard to fully grasp the complete idea of a tesseract. Cause it does not just stop at a tesseract, there is an infinite number of dimensions, which means an infinite number of cubes.

One thing that really stood out to me in this website and made it a little easier to undertsand a tesseract is this quote: "A square is a 2D shape; so, each of its corners has 2 lines coming off it at 90 degrees to each other. A cube is 3D, so each corner has 3 lines coming off it. Similarly, a tesseract is a 4D shape, so every corner has 4 lines coming off it". This made it easier to understand how we got the image of a tesseract and how its possible to create cubes within more dimensions.

https://www.scienceabc.com/pure-sciences/what-exactly-is-a-tesseract-real-life-geometry-4-dimensional.html

https://www.researchgate.net/figure/N-dimensional-Hypercube-Petrie-polygon-Orthographic-projections-from-n-1-up-to-n-8_fig8_268686702